Numerical Methods for Partial Differential Equations: A Comprehensive Exploration

Partial differential equations (PDEs) are mathematical equations that describe the behavior of physical systems that vary in space and time. They arise in a wide range of applications, including fluid dynamics, heat transfer, and solid mechanics. Solving PDEs analytically is often difficult or impossible, so numerical methods must be used to obtain approximate solutions.

Numerical Methods for Partial Differential Equations: Finite Difference and Finite Volume Methods

by Baby Professor

5 out of 5

Language	:	English
File size	:	38163 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	462 pages
X-Ray for textbooks	:	Enabled

FREE

Numerical methods for PDEs are computational techniques that use discrete approximations to represent the continuous solution of the PDE. The most common numerical methods for PDEs are the finite element method, the finite volume method, and the finite difference method.

Finite Element Method

The finite element method (FEM) is a numerical method for solving PDEs that uses a mesh of elements to represent the solution domain. The elements are typically triangles or quadrilaterals in two dimensions, or tetrahedra or hexahedra in three dimensions. The solution is approximated by a set of basis functions that are defined on the elements.

The FEM is a powerful tool for solving PDEs because it can be used to solve a wide variety of problems, including problems with complex geometries and boundary conditions. However, the FEM can be computationally expensive for large problems.

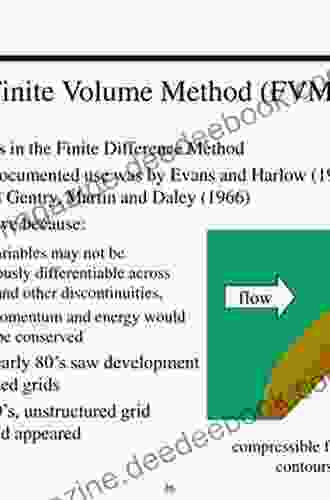
Finite Volume Method

The finite volume method (FVM) is a numerical method for solving PDEs that uses a mesh of control volumes to represent the solution domain. The control volumes are typically cells in a Cartesian grid. The solution is approximated by a set of values at the centers of the control volumes.

The FVM is a robust and efficient method for solving PDEs. It is particularly well-suited for problems involving convection and diffusion. However, the FVM can be difficult to apply to problems with complex geometries.

Finite Difference Method

The finite difference method (FDM) is a numerical method for solving PDEs that uses a mesh of points to represent the solution domain. The solution is approximated by a set of values at the points in the mesh.

The FDM is a simple and efficient method for solving PDEs. However, it can be difficult to apply to problems with complex geometries. Additionally, the FDM can be less accurate than the FEM or FVM for problems involving convection and diffusion.

Applications of Numerical Methods for PDEs

Numerical methods for PDEs are used in a wide range of applications, including:

* Computational fluid dynamics (CFD) * Heat transfer * Solid mechanics * Electromagnetism * Acoustics

CFD is used to simulate the flow of fluids. Heat transfer is used to simulate the transfer of heat. Solid mechanics is used to simulate the behavior of solids. Electromagnetism is used to simulate the behavior of electromagnetic fields. Acoustics is used to simulate the propagation of sound waves.

Numerical methods for PDEs are powerful tools that can be used to solve a wide range of problems in science and engineering. The most common numerical methods for PDEs are the finite element method, the finite volume method, and the finite difference method. Each of these methods has its own strengths and weaknesses, and the choice of method depends on the specific problem being solved.

Numerical Methods for Partial Differential Equations: Finite Difference and Finite Volume Methods

by Baby Professor

5 out of 5

Language	:	English
File size	:	38163 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	462 pages
X-Ray for textbooks	:	Enabled

FREE